Why does the formula 234/f work?
The formula 234/f is a handy way to estimate the size of a ¼ wave antenna element based on the desired frequency for the antenna. Here is how to derive that number using only other constants and conversion ratios.
How big is a ¼ wave for a given frequency? We can use the band name as a hint (14 MHz is called 20m) but we need a more exact size.
The speed of light in a vacuum (c) is 3 x 108 meters per second. The wavelength (λ) for a given frequency (usually referred by ν, but we’ll call f) is defined to be c/λ. Wavelength is the speed of light divided by the frequency. So, the wavelength in meters is:
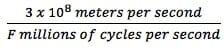
We only want to know the size of a single cycle for this frequency so we can divide both the numerator and denominator by a million. That makes the numbers much smaller! Wavelength in meters is now:
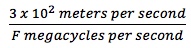
That is, wavelengths in meters uses this simple formula:
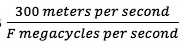
But, we want this in feet (because we’re Americans) so we need to convert meters to feet. One meter is about 3.28 feet. So, convert wavelengths to feet:
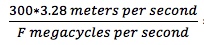
which is
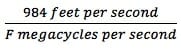
But we don’t want a whole wavelength; we want just a quarter of that for our dipole arm. Divide the whole thing by four.
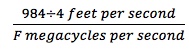
which gives us
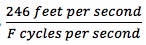
Why is this 246 and not 234? This is because we are using the speed of light as the speed of the current in the wire. But, nothing goes as fast as light but light! We need to put in the velocity factor for the wire (which is relative to the speed of light) to know how big a quarter wave is in the wire.
Choose 0.95 as the velocity factor as a guess for most antenna wire. That is, the charge propagates down the wire at about 95% of the speed of light.
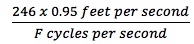
which gives us the formula
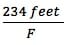
The formula 234/f isn’t “magic”; it is just a distillation of the simple formula speed-of-light/frequency with all the unit conversions and velocity factor assumptions baked-in.
How big is a ¼ wave for a given frequency? We can use the band name as a hint (14 MHz is called 20m) but we need a more exact size.
The speed of light in a vacuum (c) is 3 x 108 meters per second. The wavelength (λ) for a given frequency (usually referred by ν, but we’ll call f) is defined to be c/λ. Wavelength is the speed of light divided by the frequency. So, the wavelength in meters is:
We only want to know the size of a single cycle for this frequency so we can divide both the numerator and denominator by a million. That makes the numbers much smaller! Wavelength in meters is now:
That is, wavelengths in meters uses this simple formula:
But, we want this in feet (because we’re Americans) so we need to convert meters to feet. One meter is about 3.28 feet. So, convert wavelengths to feet:
which is
But we don’t want a whole wavelength; we want just a quarter of that for our dipole arm. Divide the whole thing by four.
which gives us
Why is this 246 and not 234? This is because we are using the speed of light as the speed of the current in the wire. But, nothing goes as fast as light but light! We need to put in the velocity factor for the wire (which is relative to the speed of light) to know how big a quarter wave is in the wire.
Choose 0.95 as the velocity factor as a guess for most antenna wire. That is, the charge propagates down the wire at about 95% of the speed of light.
which gives us the formula
The formula 234/f isn’t “magic”; it is just a distillation of the simple formula speed-of-light/frequency with all the unit conversions and velocity factor assumptions baked-in.
May 15, 2018


